GENERALIZED LOB'S THEOREM STRONG REFLECTION PRINCIPLES AND LARGE CARDINAL AXIOMS CONSISTENCY RESULTS IN TOPOLOGY
1 Israel Institute of Technology, Haifa, Israel
ABSTRACT

Keywords: ![]()
ARTICLE HISTORY: Received: 24 May 2017, Revised: 13 June 2017, Accepted: 18 July 2017, Published: 9 August 2017
Contribution/ Originality: This study uses new estimation methodology in forecasting the daily precipitation by using different weather parameters for two regions at United states of America. The study can be considered as a comparison between the two well-known methods which are artificial networks and Adaptive Neuro-Fuzzy Inference System.
1. INTRODUCTION


Remark 1.3.We remind that in Henkin semantics, each sort of second-order variable has a particular domain of its own to range over, which may be a proper subset of all sets or functions of that sort. Henkin [2] defined these semantics and proved that Gödel's completeness theorem and compactness theorem, which hold for first-order logic, carry over to second-order logic with Henkin semantics. This is because Henkin semantics are almost identical to many-sorted first-order semantics, where additional sorts of variables are added to simulate the new variables of second-order logic. Second-order logic with Henkin semantics is not more expressive than first-order logic. Henkin semantics are commonly used in the study of second-order arithmetic. Vaananen [6] argued that the choice between Henkin models and full models for second-order logic is analogous to the choice between ZFC and V as a basis for set theory: "As with second-order logic, we cannot really choose whether we axiomatize mathematics using V or ZFC. The result is the same in both cases, as ZFC is the best attempt so far to use V as an axiomatization of mathematics."
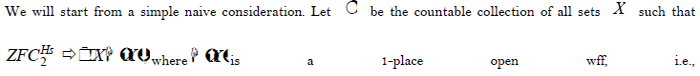








2. DERIVATION INCONSISTENT COUNTABLE SET IN 


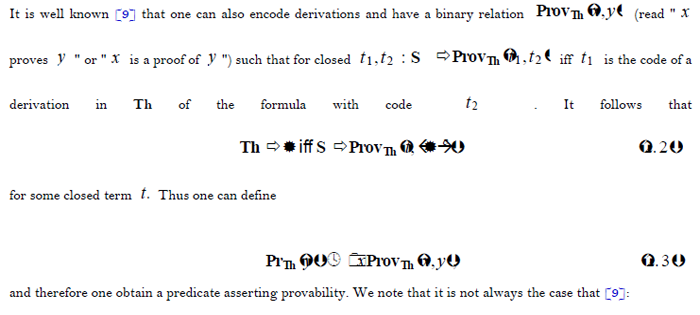
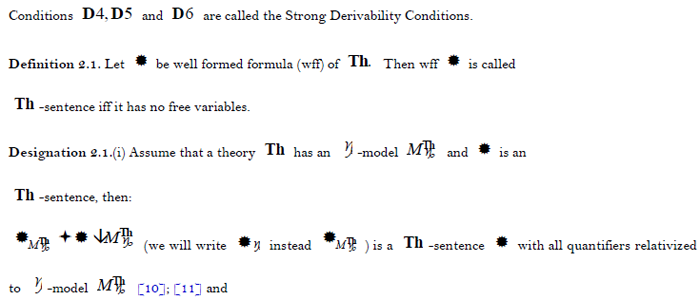

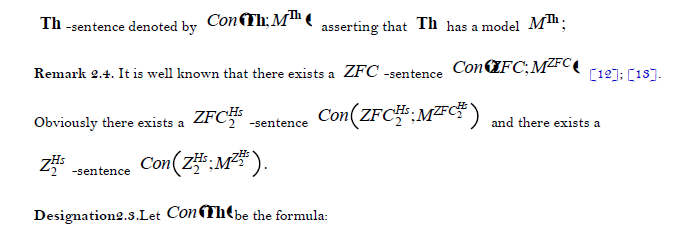



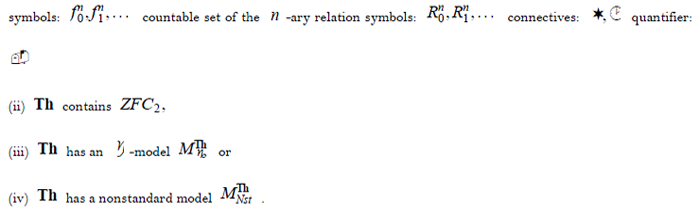

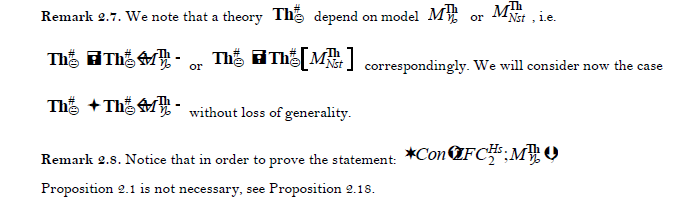




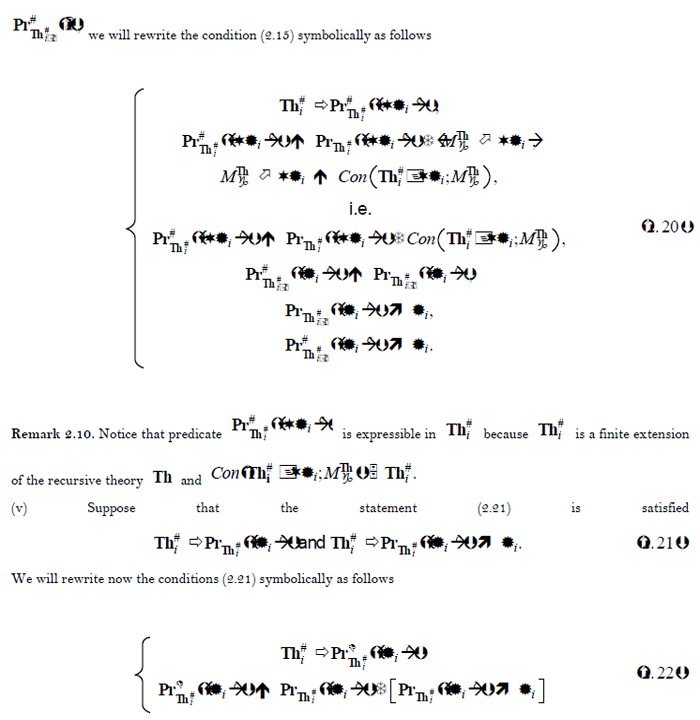
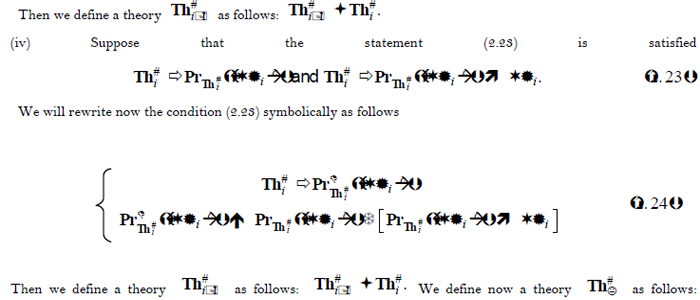

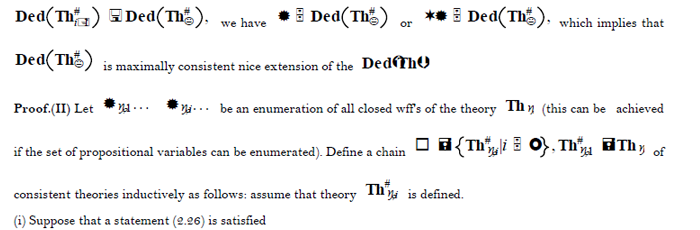

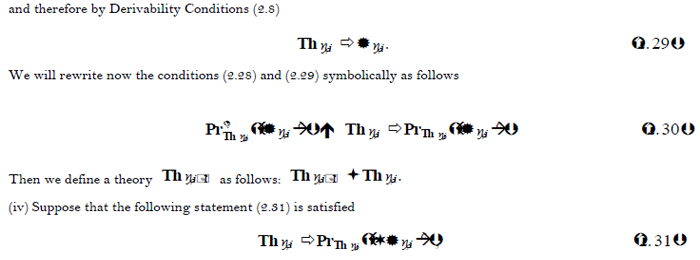



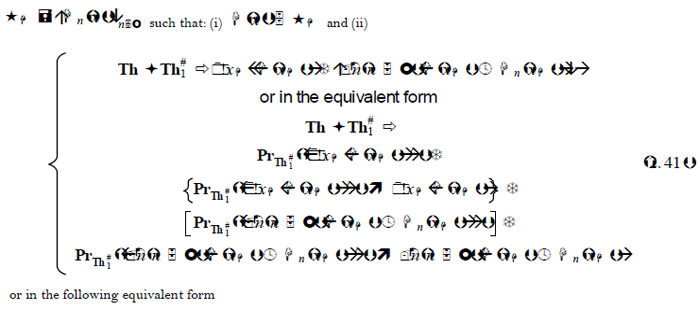




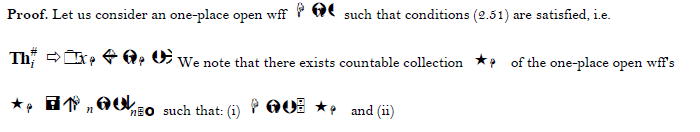
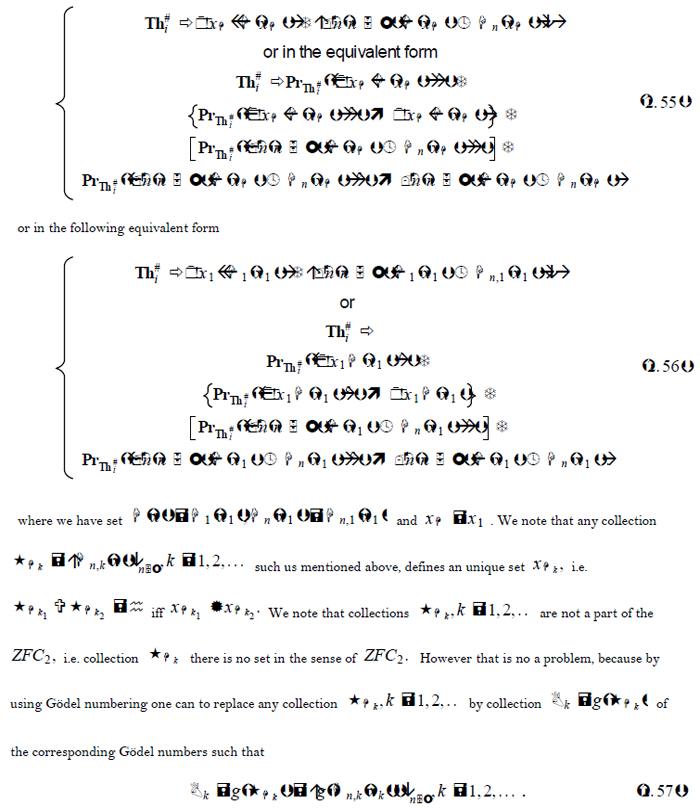


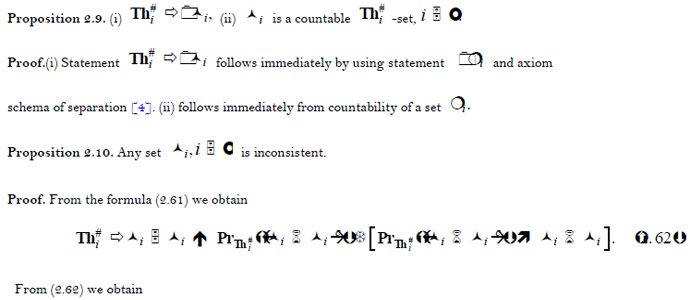

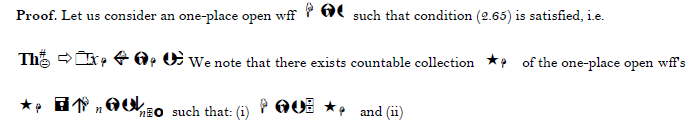
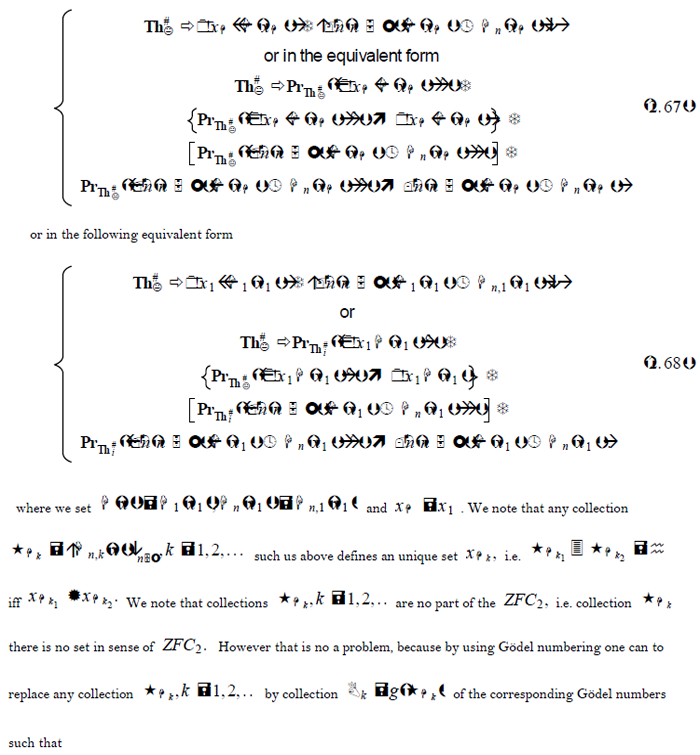


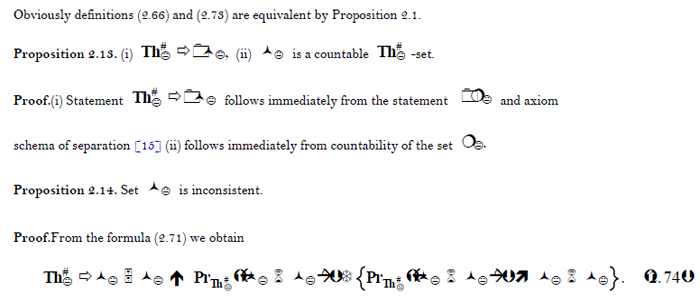

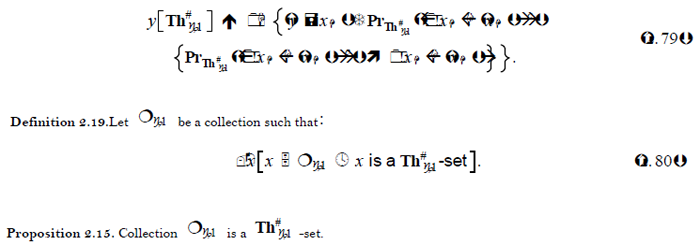









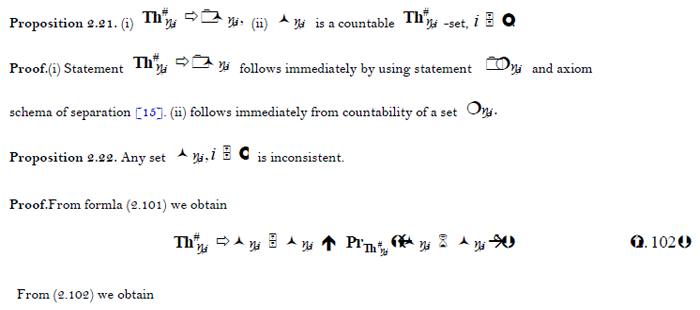











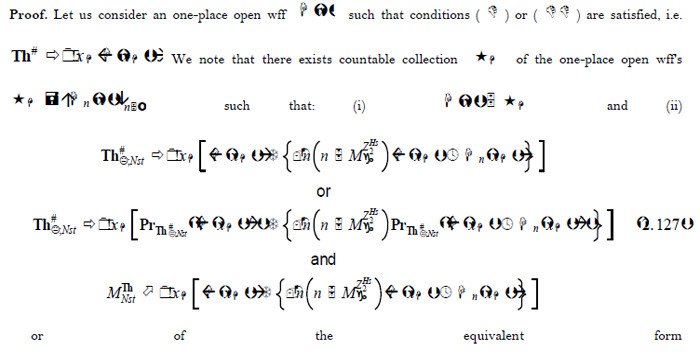



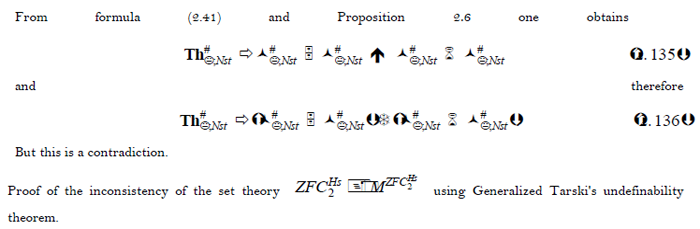


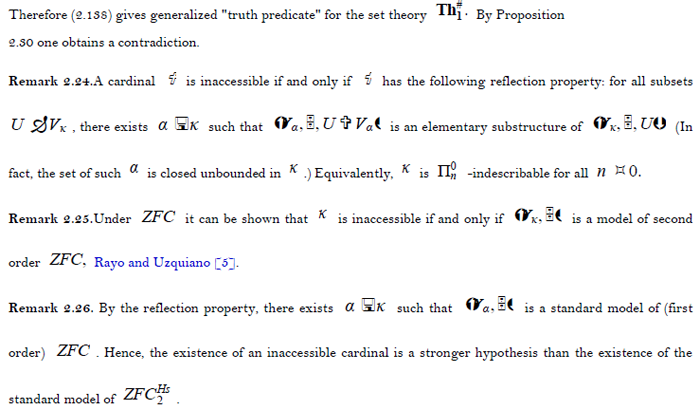
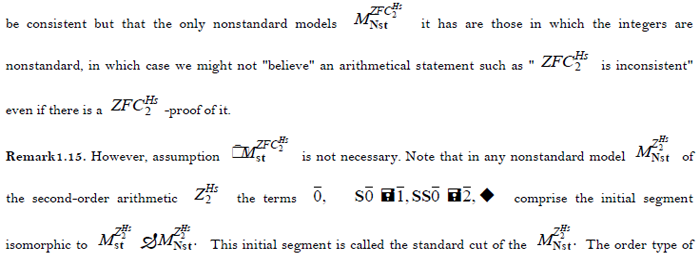
3. DERIVATION INCONSISTENT COUNTABLE SET IN SET THEORY ZFC2 WITH THE FULL SEMANTICS








4. CONSISTENCY RESULTS IN TOPOLOGY



5. CONCLUSION

Funding: This study received no specific financial support. |
Competing Interests: The authors declare that they have no competing interests. |
Contributors/Acknowledgement: All authors contributed equally to the conception and design of the study. |
REFERENCES
[1] E. Nelson, "Warning signs of a possible collapse of contemporary mathematics. Retrieved from https://web.math.princeton.edu/~nelson/papers/warn.pdf," n.d.
[2] L. Henkin, "Completeness in the theory of types," Journal of Symbolic Logic, vol. 15, pp. 81--91, 1950. View at Google Scholar | View at Publisher
[3] M. Rossberg, First-order logic, second-order logic, and completeness. In V.Hendricks et al., (Eds)., First-order logic revisited. Berlin: Logos-Verlag, 2004.
[4] S. Shapiro, Foundations without foundationalism: A case for second-order logic: Oxford University Press, 2000.
[5] A. Rayo and G. Uzquiano, "Toward a theory of second-order consequence," Notre Dame Journal of Formal Logic, vol. 40, pp. 315-325, 1999. View at Google Scholar | View at Publisher
[6] J. Vaananen, "Second-order logic and foundations of mathematics," Bulletin of Symbolic Logic, vol. 7, pp. 504-520, 2001. View at Google Scholar | View at Publisher
[7] A. Bovykin, "On order-types of models of arithmetic," Ph.D. Thesis , University of Birmingham. pp:109, 2000.
[8] J. Foukzon, "Strong reflection principles and large cardinal axioms," presented at the Fall Southeastern Sectional Meeting University of Louisville, Louisville, KY October 5-6, 2013 (Saturday -Sunday) Meeting #1092, 2013.
[9] C. Smorynski, Handbook of mathematical logic, Edited by J. Barwise: North-Holland Publishing Company, 1977.
[10] J. Foukzon and E. R. Men'kova, "Generalized löb's theorem. Strong reflection principles and large cardinal axioms," Advances in Pure Mathematics, vol. 3, pp. 368-373, 2013.View at Publisher
[11] P. Lindstrom, "First order predicate logic with generalized quantifiers," Theoria, vol. 32, pp. 186-195, 1966. View at Google Scholar | View at Publisher
[12] F. Larusson, "A proof that cannot be formalized in ZFC. Fri Oct 5 23:35:03 EDT." Retrieved from http://www.cs.nyu.edu/pipermail/fom/2007-October/012009.html, 2007.
[13] P. J. Cohen, Set theory and the continuum hypothesis: Courier Corporation, 2008.
[14] E. Mendelson, Introduction to mathematical logic: Springer 1997-06-01, 1997.
[15] G. Takeuti and W. M. Zaring, Introduction to axiomatic set theory: Springer-Verlag, 1971.
[16] J. Foukzon, "Generalized lob's theorem. Strong reflection principles and large cardinal axioms," presented at the International Conference on Topology and its Applications, July 3-7, 2014, Nafpaktos, Greece, 2014.
[17] F. D. Tall, "On the cardinality of lindelöf spaces with points," Topology and its Applications, vol. 63, pp. 21--38, 1995. View at Google Scholar | View at Publisher
[18] R. R. Dias and F. D. Tall, "Instituto de matemática e estatística universidade de São Paulo,15-th Galway Topology Colloquium, Oxford," 2012.
[19] J. Foukzon, "Strong reflection principles and large cardinal axioms," Pure and Applied Mathematics Journal, vol. 2, pp. 119-127, 2013. View at Publisher
[20] J. Foukzon, "An possible generalization of the lob's theorem," presented at the AMS Sectional Meeting AMS Special Session, Spring Western Sectional Meeting University of Colorado Boulder, Boulder, CO 13-14 April 2013. Meeting # 1089, 2013.
[21] J. Foukzon, "Consistency results in topology and homotopy theory," Pure and Applied Mathematics Journal, vol. 4, pp. 1-5, 2015.View at Google Scholar | View at Publisher
[22] J. Foukzon, "Generalized lob's theorem. Strong reflection principles and large cardinal axioms," presented at the IX Iberoamerican Conference on Topology and its Applications 24-27 June, 2014 Almeria, Spain, 2014.
[23] J. Foukzon, "Generalized lob's theorem. Strong reflection principles and large cardinal axioms," presented at the International Conference on Topology and its Applications, July 3-7, 2014, Nafpaktos, Greece, 2014.






